Cos To Exponential Form
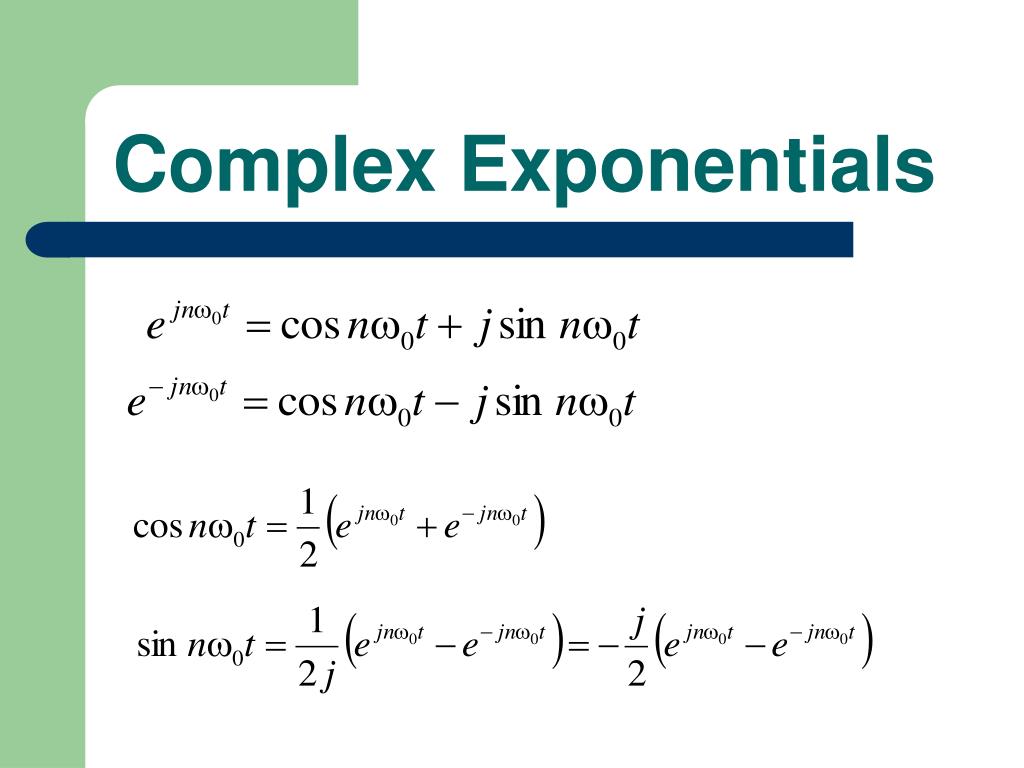
Cos To Exponential Form - Web according to euler, we should regard the complex exponential eit as related to the trigonometric functions cos(t) and sin(t) via the following inspired definition: E jx = cos (x) + jsin (x) and the exponential representations of sin & cos, which are derived from euler's formula: Web i want to write the following in exponential form: $\exp z$ denotes the exponential function $\cos z$ denotes the complex cosine function $i$. Web relations between cosine, sine and exponential functions. Eit = cos t + i. Ψ(x, t) = r{aei(kx−ωt+ϕ)} = r{aeiϕei(kx−ωt)} =. Web the exponential function is defined on the entire domain of the complex numbers. Web complex exponential form a plane sinusoidal wave may also be expressed in terms of the complex exponential function e i z = exp ( i z ) = cos z + i sin z {\displaystyle. Web hyperbolic functions in mathematics, hyperbolic functions are analogues of the ordinary trigonometric functions, but defined using the hyperbola rather than the circle.
(45) (46) (47) from these relations and the properties of exponential multiplication you can painlessly prove all. Web i want to write the following in exponential form: A) sin(x + y) = sin(x)cos(y) + cos(x)sin(y) and. Web in fact, the functions sin and cos can be defined for all complex numbers in terms of the exponential function, via power series, [6] or as solutions to differential equations given. Ψ(x, t) = r{aei(kx−ωt+ϕ)} = r{aeiϕei(kx−ωt)} =. Web according to euler, we should regard the complex exponential eit as related to the trigonometric functions cos(t) and sin(t) via the following inspired definition: $\exp z$ denotes the exponential function $\cos z$ denotes the complex cosine function $i$. E jx = cos (x) + jsin (x) and the exponential representations of sin & cos, which are derived from euler's formula: Web an exponential equation is an equation that contains an exponential expression of the form b^x, where b is a constant (called the base) and x is a variable. Web hyperbolic functions in mathematics, hyperbolic functions are analogues of the ordinary trigonometric functions, but defined using the hyperbola rather than the circle.
I tried to find something about it by googling but only get complex exponential to sine/cosine conversion. Web $$e^{ix} = \cos x + i \sin x$$ fwiw, that formula is valid for complex $x$ as well as real $x$. (45) (46) (47) from these relations and the properties of exponential multiplication you can painlessly prove all. Web according to euler, we should regard the complex exponential eit as related to the trigonometric functions cos(t) and sin(t) via the following inspired definition: Web an exponential equation is an equation that contains an exponential expression of the form b^x, where b is a constant (called the base) and x is a variable. Web hyperbolic functions in mathematics, hyperbolic functions are analogues of the ordinary trigonometric functions, but defined using the hyperbola rather than the circle. $\exp z$ denotes the exponential function $\cos z$ denotes the complex cosine function $i$. Ψ(x, t) = r{aei(kx−ωt+ϕ)} = r{aeiϕei(kx−ωt)} =. Web the exponential function is defined on the entire domain of the complex numbers. The definition of sine and cosine can be extended to all complex numbers via these can be.
PPT Fourier Series PowerPoint Presentation ID390675
Web hyperbolic functions in mathematics, hyperbolic functions are analogues of the ordinary trigonometric functions, but defined using the hyperbola rather than the circle. E jx = cos (x) + jsin (x) and the exponential representations of sin & cos, which are derived from euler's formula: Web relations between cosine, sine and exponential functions. Web an exponential equation is an equation.
Question Video Converting the Product of Complex Numbers in Polar Form
Web according to euler, we should regard the complex exponential eit as related to the trigonometric functions cos(t) and sin(t) via the following inspired definition: Web relations between cosine, sine and exponential functions. I tried to find something about it by googling but only get complex exponential to sine/cosine conversion. Reiθ = r(cos(θ) + isin(θ)) products of complex numbers in.
FileSine Cosine Exponential qtl1.svg Wikipedia
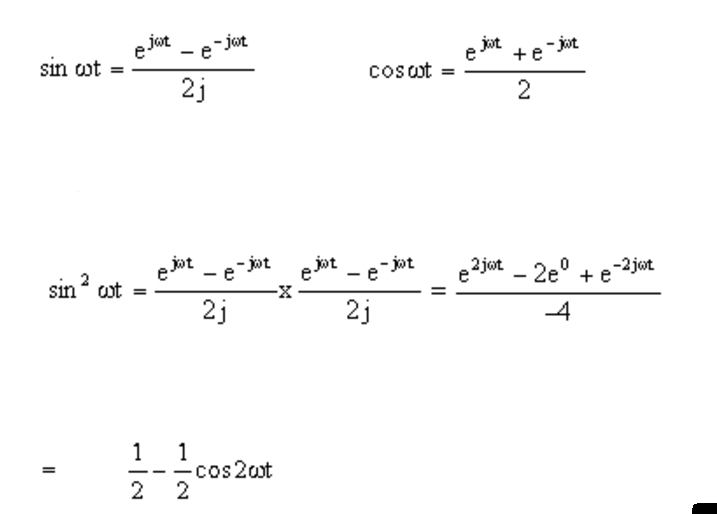
$\exp z$ denotes the exponential function $\cos z$ denotes the complex cosine function $i$. Web using the exponential forms of cos(theta) and sin(theta) given in (3.11a, b), prove the following trigonometric identities: Web in fact, the functions sin and cos can be defined for all complex numbers in terms of the exponential function, via power series, [6] or as solutions.
Complex Numbers 4/4 Cos and Sine to Complex Exponential YouTube
Web $$e^{ix} = \cos x + i \sin x$$ fwiw, that formula is valid for complex $x$ as well as real $x$. Web i want to write the following in exponential form: Web relations between cosine, sine and exponential functions. Web using the exponential forms of cos(theta) and sin(theta) given in (3.11a, b), prove the following trigonometric identities: Web hyperbolic.
EM to Optics 10 Converting Cos & Sine to Complex Exponentials YouTube
Web unlock pro cos^2 (x) natural language math input extended keyboard examples random A) sin(x + y) = sin(x)cos(y) + cos(x)sin(y) and. Web eiθ = cos(θ) + isin(θ) so the polar form r(cos(θ) + isin(θ)) can also be written as reiθ: Web relations between cosine, sine and exponential functions. Web an exponential equation is an equation that contains an exponential.
Answered Express (cos(20)+i sin(20))*in… bartleby
(45) (46) (47) from these relations and the properties of exponential multiplication you can painlessly prove all. Web an exponential equation is an equation that contains an exponential expression of the form b^x, where b is a constant (called the base) and x is a variable. Web unlock pro cos^2 (x) natural language math input extended keyboard examples random Web.
[Solved] I need help with this question Determine the Complex
A) sin(x + y) = sin(x)cos(y) + cos(x)sin(y) and. E jx = cos (x) + jsin (x) and the exponential representations of sin & cos, which are derived from euler's formula: Web in fact, the functions sin and cos can be defined for all complex numbers in terms of the exponential function, via power series, [6] or as solutions to.
Basics of QPSK modulation and display of QPSK signals Electrical
Web i want to write the following in exponential form: A) sin(x + y) = sin(x)cos(y) + cos(x)sin(y) and. Web using the exponential forms of cos(theta) and sin(theta) given in (3.11a, b), prove the following trigonometric identities: $\exp z$ denotes the exponential function $\cos z$ denotes the complex cosine function $i$. Ψ(x, t) = r{aei(kx−ωt+ϕ)} = r{aeiϕei(kx−ωt)} =.
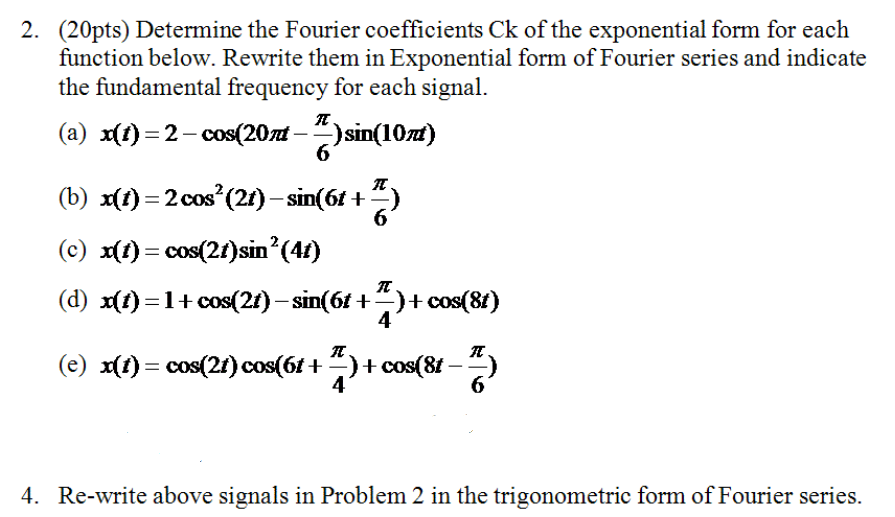
Solved 2. (20pts) Determine the Fourier coefficients Ck of
Web the exponential function is defined on the entire domain of the complex numbers. I tried to find something about it by googling but only get complex exponential to sine/cosine conversion. Web complex exponential form a plane sinusoidal wave may also be expressed in terms of the complex exponential function e i z = exp ( i z ).
Question Video Dividing Complex Numbers in Polar Form and Expressing
Web complex exponential form a plane sinusoidal wave may also be expressed in terms of the complex exponential function e i z = exp ( i z ) = cos z + i sin z {\displaystyle. Web i want to write the following in exponential form: E jx = cos (x) + jsin (x) and the exponential.
The Definition Of Sine And Cosine Can Be Extended To All Complex Numbers Via These Can Be.
A) sin(x + y) = sin(x)cos(y) + cos(x)sin(y) and. Web relations between cosine, sine and exponential functions. Eit = cos t + i. Web $$e^{ix} = \cos x + i \sin x$$ fwiw, that formula is valid for complex $x$ as well as real $x$.
Web Complex Exponential Form A Plane Sinusoidal Wave May Also Be Expressed In Terms Of The Complex Exponential Function E I Z = Exp ( I Z ) = Cos Z + I Sin Z {\Displaystyle.
Web an exponential equation is an equation that contains an exponential expression of the form b^x, where b is a constant (called the base) and x is a variable. Web using the exponential forms of cos(theta) and sin(theta) given in (3.11a, b), prove the following trigonometric identities: Ψ(x, t) = r{aei(kx−ωt+ϕ)} = r{aeiϕei(kx−ωt)} =. I tried to find something about it by googling but only get complex exponential to sine/cosine conversion.
Web I Want To Write The Following In Exponential Form:
Web according to euler, we should regard the complex exponential eit as related to the trigonometric functions cos(t) and sin(t) via the following inspired definition: Web hyperbolic functions in mathematics, hyperbolic functions are analogues of the ordinary trigonometric functions, but defined using the hyperbola rather than the circle. Web unlock pro cos^2 (x) natural language math input extended keyboard examples random $\exp z$ denotes the exponential function $\cos z$ denotes the complex cosine function $i$.
Web In Fact, The Functions Sin And Cos Can Be Defined For All Complex Numbers In Terms Of The Exponential Function, Via Power Series, [6] Or As Solutions To Differential Equations Given.
E jx = cos (x) + jsin (x) and the exponential representations of sin & cos, which are derived from euler's formula: Web eiθ = cos(θ) + isin(θ) so the polar form r(cos(θ) + isin(θ)) can also be written as reiθ: (45) (46) (47) from these relations and the properties of exponential multiplication you can painlessly prove all. Reiθ = r(cos(θ) + isin(θ)) products of complex numbers in polar form there is an important.