Reduced Row Echelon Form Steps
Reduced Row Echelon Form Steps - This will eliminate the first entry of row 2. Understand how to perform elementary row operations. All entries below a leading entry are zero. A matrix is in row echelon form if it meets the following requirements: Unlike the row echelon form, the reduced row echelon form of a matrix is unique and does not depend on the algorithm used to compute it. Web reduced row echelon form is at the other end of the spectrum; Learning math takes practice, lots of practice. 4.the leading entry in each nonzero row is 1. Web reduced row echelon form steven bellenot may 11, 2008 reduced row echelon form { a.k.a. Web and as stated earlier, there are only three row reducing techniques:
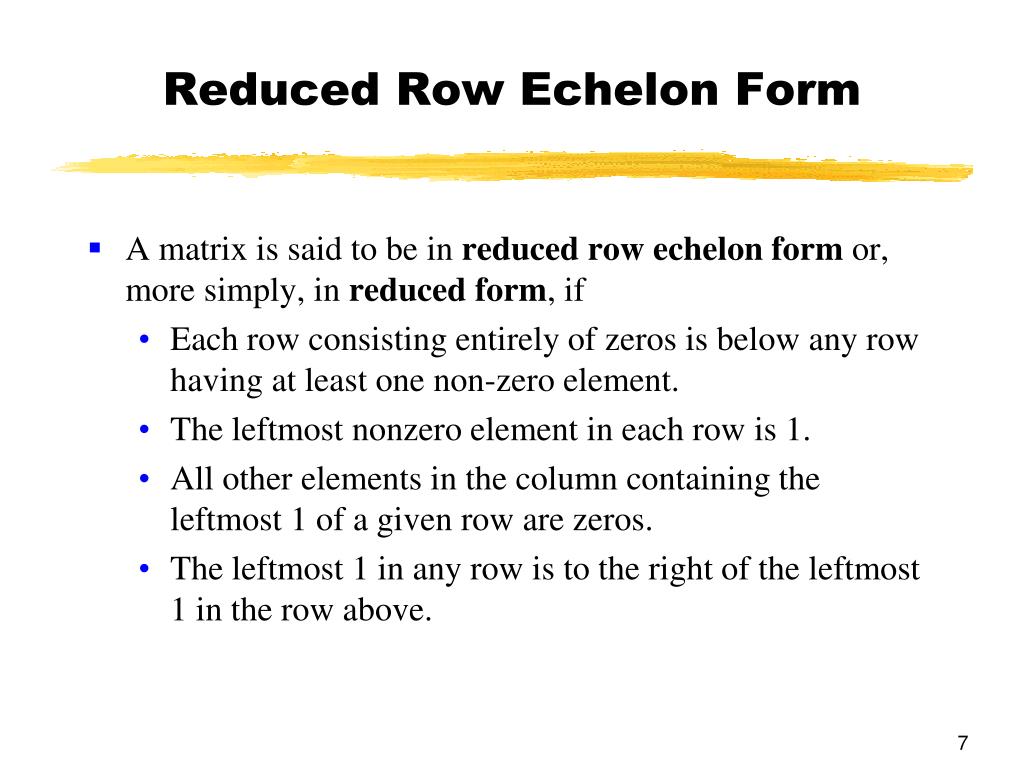
The leading entry in each nonzero row is 1. Web reduced row echelon form steven bellenot may 11, 2008 reduced row echelon form { a.k.a. Web solution theorem 1.2.2: Questions tips & thanks sort by: Switch row 1 and row 3. Web a matrix is said to be in reduced row echelon form when it is in row echelon form and its basic columns are vectors of the standard basis (i.e., vectors having one entry equal to 1 and all the other entries equal to 0). Unlike the row echelon form, the reduced row echelon form of a matrix is unique and does not depend on the algorithm used to compute it. Web to solve this system, the matrix has to be reduced into reduced echelon form. A matrix is in row echelon form if it meets the following requirements: • ( 44 votes) flag tim 10 years ago
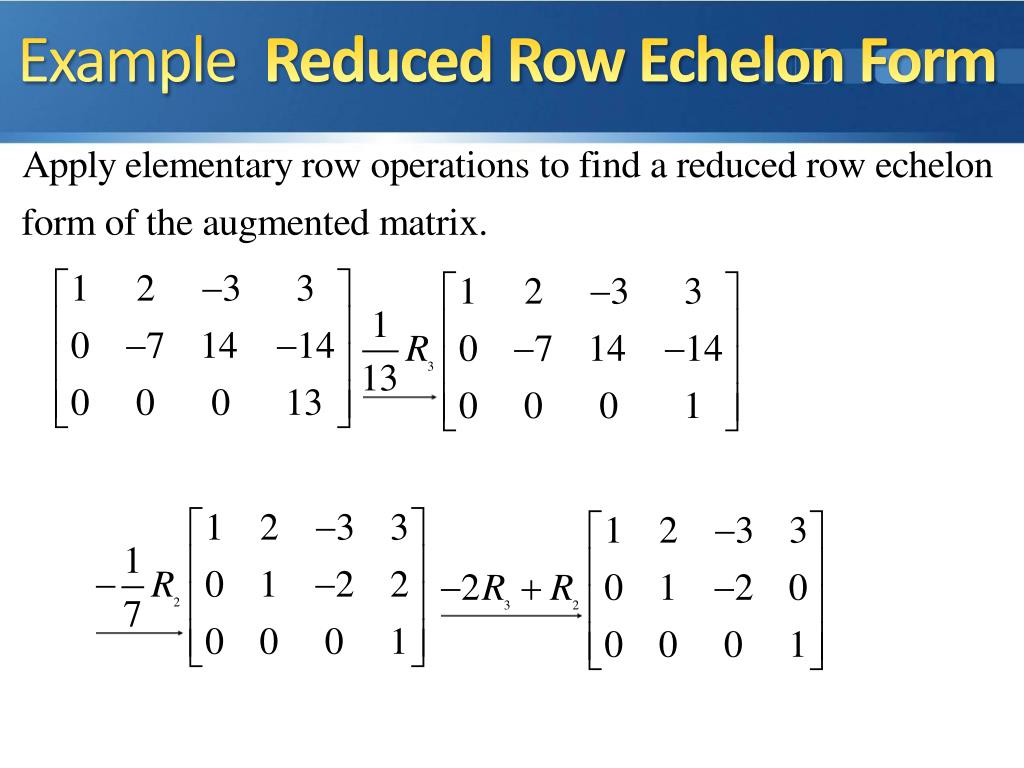
You can enter a matrix manually into the following form or paste a whole matrix at once, see details below. Web we write the reduced row echelon form of a matrix a as rref ( a). In each of the remaining rows, the element located below the pivot becomes 0 by adding the first multiplied by the. We perform one more step to obtain the reduced echelon form. The first nonzero entry in a row. The leading entry in each row is 1. Web is there a step by step calculator for math? Beginning with the rightmost pivot and working upward and to the left, create zeros. Advanced math questions and answers. Rref for some reason our text fails to de ne rref (reduced row echelon form) and so we de ne it here.
PPT Chapter 4 Systems of Linear Equations; Matrices PowerPoint
If a is an invertible square matrix, then rref ( a) = i. Symbolab is the best step by step calculator for a wide range of math problems, from basic arithmetic to advanced calculus and linear algebra. The calculator will find the row echelon form (rref) of the given augmented matrix for a given field, like real numbers (r), complex.
Reduced Row Echelon Form Matrix Calculator CALCKP
The leading one in a nonzero row appears to the left of the leading one in any lower row. Instead of gaussian elimination and back substitution, a system of equations can be solved by bringing a. It shows you the solution, graph, detailed steps and explanations for each problem. Questions tips & thanks sort by: Web solution theorem 1.2.2:
Transforming Square Matrices Into Reduced Row Echelon Form 7 Steps
5.each leading 1 is the only nonzero entry in its column. Multiply row 2 by 3 and row 3. Web solution theorem 1.2.2: Unlike the row echelon form, the reduced row echelon form of a matrix is unique and does not depend on the algorithm used to compute it. It shows you the solution, graph, detailed steps and explanations for.
2.3 Reduced Row Echelon Form YouTube
Nonzero rows appear above the zero rows. In other words, subtract row 1 from row 2. Web reduced row echelon form is at the other end of the spectrum; In each of the remaining rows, the element located below the pivot becomes 0 by adding the first multiplied by the. Rows of all zeros, if any, are grouped at the.
Reduced Row Echelon Form Definition definitionyoi
Web to solve this system, the matrix has to be reduced into reduced echelon form. Web reduced row echolon form calculator. Symbolab is the best step by step calculator for a wide range of math problems, from basic arithmetic to advanced calculus and linear algebra. It shows you the solution, graph, detailed steps and explanations for each problem. What is.
linear algebra Understanding the definition of row echelon form from
Unlike the row echelon form, the reduced row echelon form of a matrix is unique and does not depend on the algorithm used to compute it. All entries below a leading entry are zero. What is row echelon form? Just like running, it takes practice and dedication. Web and as stated earlier, there are only three row reducing techniques:
Uniqueness of Reduced Row Echelon Form YouTube
The leading entry in each nonzero row is 1. Web how to find reduced row echelon form we can start by getting a pivot 1 in the first row. 5.each leading 1 is the only nonzero entry in its column. A system with many solutions solution objectives learn to replace a system of linear equations by an augmented matrix. Web.
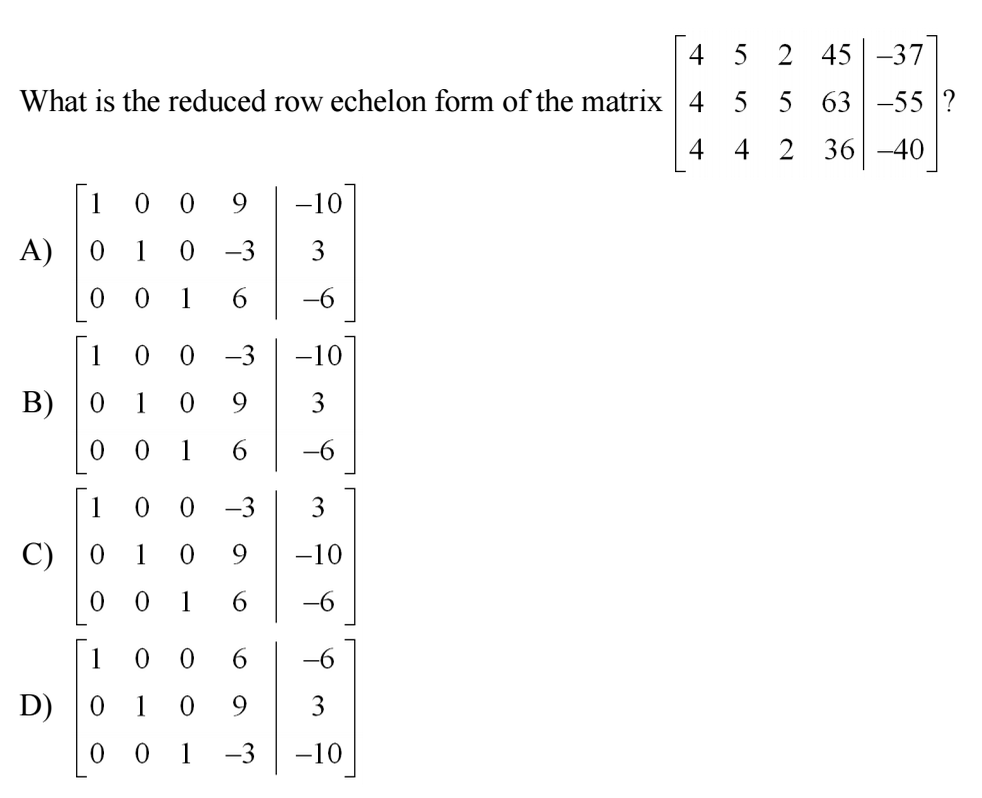
Solved What is the reduced row echelon form of the matrix
In other words, subtract row 1 from row 2. All entries below a leading entry are zero. Web to solve this system, the matrix has to be reduced into reduced echelon form. We perform one more step to obtain the reduced echelon form. (2.1) use the reduced row echelon form to verify that det (−a)=det (−at).
PPT Multivariate Linear Systems and Row Operations PowerPoint
We perform one more step to obtain the reduced echelon form. Switch row 1 and row 3. A system with many solutions solution objectives learn to replace a system of linear equations by an augmented matrix. Symbolab is the best step by step calculator for a wide range of math problems, from basic arithmetic to advanced calculus and linear algebra..
Row Reduced echelon form YouTube
For a matrix to be in rref every leading (nonzero) coefficient must be 1. The row echelon form of an inconsistent system example 1.2.8: Nonzero rows appear above the zero rows. In each of the remaining rows, the element located below the pivot becomes 0 by adding the first multiplied by the. You can enter a matrix manually into the.
You Can Enter A Matrix Manually Into The Following Form Or Paste A Whole Matrix At Once, See Details Below.
Web solution theorem 1.2.2: A=⎣⎡32−1110−2−1−2⎦⎤ (2.2) use inspection to find (without calculating the determinant) ∣∣100001000010000−2∣∣ (2.3) use inspection to find (without calculating the determinant) ∣∣. The leading one in a nonzero row appears to the left of the leading one in any lower row. Beginning with the rightmost pivot and working upward and to the left, create zeros.
Understand How To Perform Elementary Row Operations.
In each of the remaining rows, the element located below the pivot becomes 0 by adding the first multiplied by the. Learning math takes practice, lots of practice. A matrix is in row echelon form if it meets the following requirements: The leading entry in each nonzero row is 1.
Symbolab Is The Best Step By Step Calculator For A Wide Range Of Math Problems, From Basic Arithmetic To Advanced Calculus And Linear Algebra.
Advanced math questions and answers. The first nonzero entry in a row. Web reduced row echelon form is at the other end of the spectrum; Rows of all zeros, if any, are grouped at the bottom.
In Any Nonzero Row, The Rst Nonzero Entry Is A One (Called The Leading One).
Questions tips & thanks sort by: Unlike the row echelon form, the reduced row echelon form of a matrix is unique and does not depend on the algorithm used to compute it. If in the first column there is some element that is not null, we. For a matrix to be in rref every leading (nonzero) coefficient must be 1.