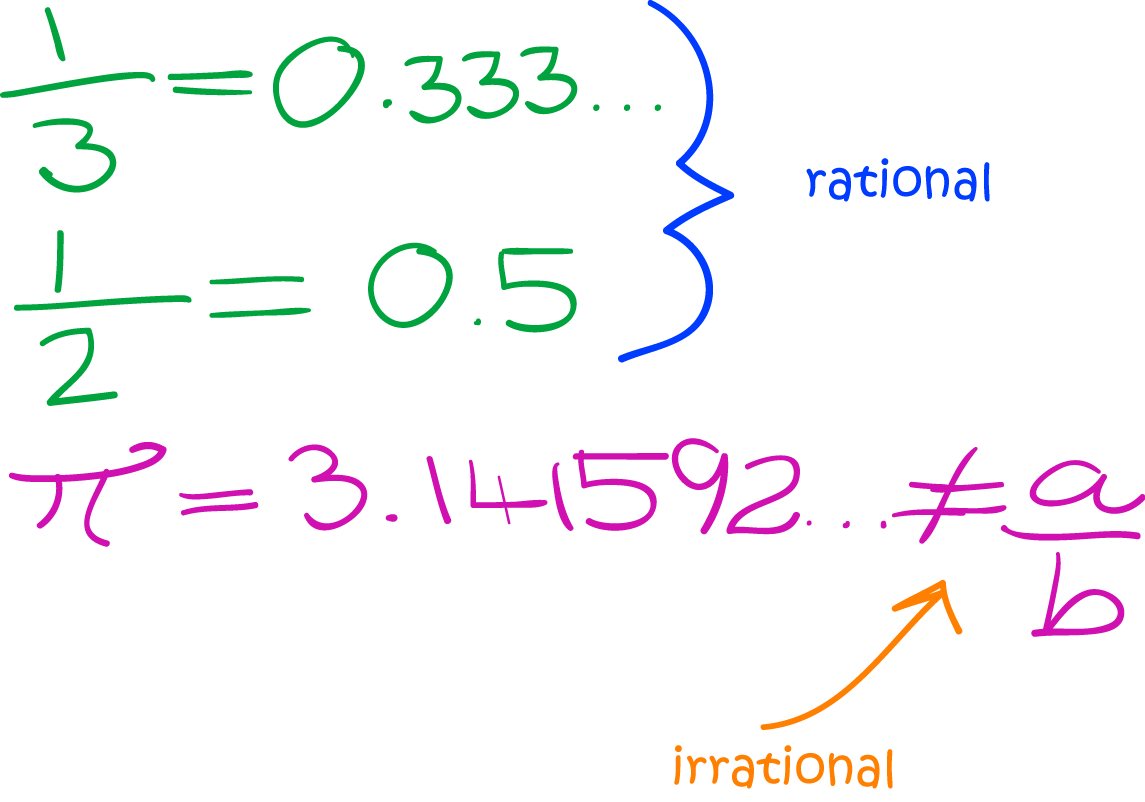Irrational Numbers Written In Decimal Form
Irrational Numbers Written In Decimal Form - The set of real numbers that cannot be written in the form of p q, where p and q are integers, is known as irrational numbers. People have calculated pi to over a quadrillion decimal places and still there is no pattern. Web another way to express an irrational number in decimal form is to make up a decimal that perhaps has some pattern to it, but never terminates or repeats. Web an irrational number is a number that cannot be written as the ratio of two integers. Web to decide if an integer is a rational number, we try to write it as a ratio of two integers. An irrational number cannot be fully written down in fraction or. An irrational number is a real number that cannot be expressed as a ratio of integers; Irrational numbers are nonterminating and nonrepeating. Let's summarize a method we can use. 3= 3 1, −8= −8 1, 0=.
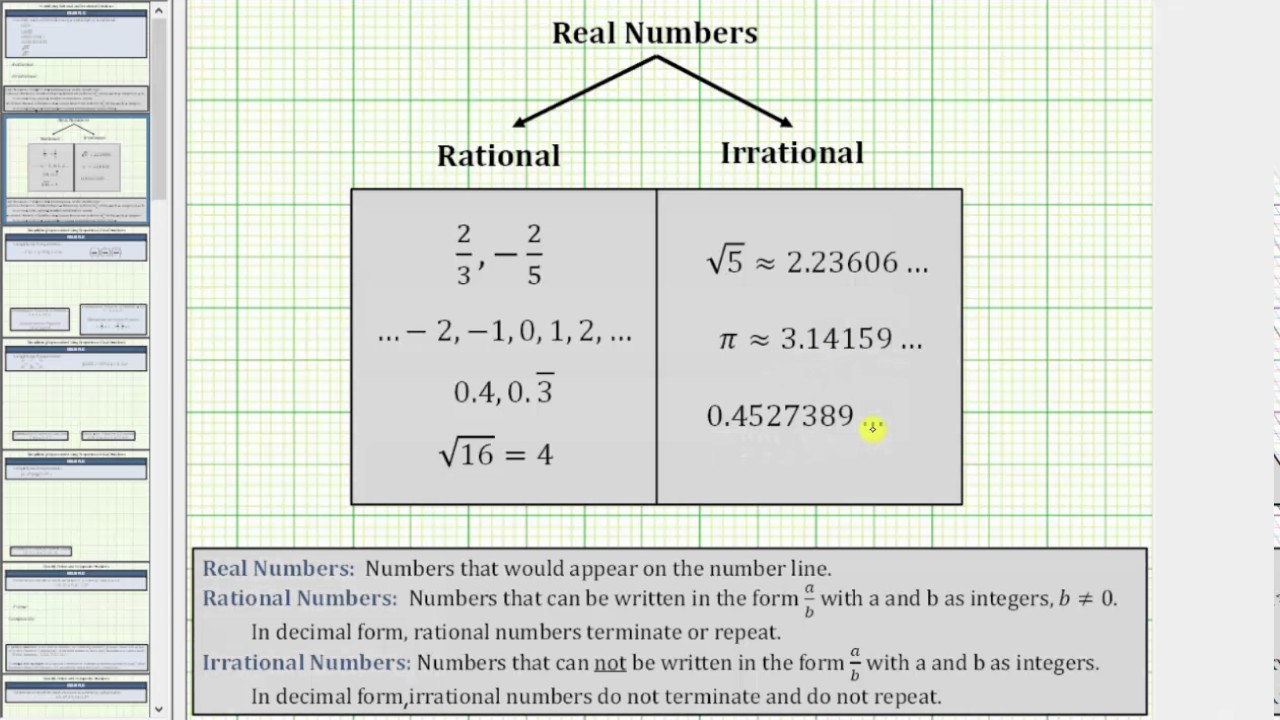
An easy way to do this is to write it as a fraction with denominator one. Web a rational number is of the form , p = numerator, q= denominator, where p and q are integers and q ≠0. Let's summarize a method we can use. Web rational numbers are numbers that can be written as a ratio of two integers. Web faqs what are irrational numbers? Web irrational numbers are numbers that can not be expressed in the form of p/q where p and q are integers and q does not equal zero. Web an irrational number is a number that cannot be written as the ratio of two integers. Web an irrational number is a number that cannot be written in the form of a common fraction of two integers. Web 1 2 3 4 5 6 rational and irrational numbers a number is described as rational if it can be written as a fraction (one integer divided by another integer). Irrational numbers can not be.
An easy way to do this is to write it as a fraction with denominator one. Web rational numbers are numbers that can be written as a ratio of two integers. Regardless of the form used, 5. The set of real numbers that cannot be written in the form of p q, where p and q are integers, is known as irrational numbers. The decimal form of a. Its decimal form does not stop and does not repeat. Web in mathematics, an irrational number is a real number that cannot be written as a complete ratio of two integers. For example, √2 is an irrational number. I verified π π and all the. Web definition of irrational numbers.
Question Video Writing a Decimal in Standard Form Nagwa
Its decimal form does not stop and does not repeat. Web pi is a famous irrational number. Web decimal expansions for rational numbers can be either terminating or repeating decimals. For example, √2 is an irrational number. So irrational number is a number that is not rational that means it is a.
(M7 Topic B) Students explore decimal expansions and irrational numbers
Web definition of irrational numbers. Web which statements are true for irrational numbers written in decimal form? Web 1 2 3 4 5 6 rational and irrational numbers a number is described as rational if it can be written as a fraction (one integer divided by another integer). Web rational numbers are numbers that can be written as a ratio.
Irrational Number Math Definitions Letter I
Web faqs what are irrational numbers? Web another way to express an irrational number in decimal form is to make up a decimal that perhaps has some pattern to it, but never terminates or repeats. Its decimal form does not stop and does not repeat. Irrational numbers are nonterminating and nonrepeating. For example, √2 is an irrational number.
M102 TB 1.4 Irrational Numbers & Decimal Representations YouTube
For example, √2 is an irrational number. Web rational numbers are numbers that can be written as a ratio of two integers. It is part of the set of real numbers alongside rational numbers. People have calculated pi to over a quadrillion decimal places and still there is no pattern. I verified π π and all the.
9 stdmath125julydecimal Form Of Irrational Number नववी गणित भाग 1
3= 3 1, −8= −8 1, 0=. Web irrational numbers are numbers that can not be expressed in the form of p/q where p and q are integers and q does not equal zero. Web rational numbers are numbers that can be written as a ratio of two integers. Web faqs what are irrational numbers? It is part of the.
PPT Rational & Irrational Numbers PowerPoint Presentation, free
Web rational numbers are numbers that can be written as a ratio of two integers. Web an irrational number is a number that cannot be written as the ratio of two integers. People have calculated pi to over a quadrillion decimal places and still there is no pattern. The first few digits look like this:. Irrational numbers are nonterminating and.
Determine Rational or Irrational Numbers (Square Roots and Decimals
Web definition of irrational numbers. Web in mathematics, an irrational number is a real number that cannot be written as a complete ratio of two integers. The first few digits look like this:. Web 1 2 3 4 5 6 rational and irrational numbers a number is described as rational if it can be written as a fraction (one integer.
Finding if Decimal Number is rational or irrational Classifiying rat
Regardless of the form used, 5. ¯ 3 is rational because this number can be written as the ratio of 16. Web an irrational number is a number that cannot be written as the ratio of two integers. 1 2, 11 3, 5 1, 3.25, 0.252525. So irrational number is a number that is not rational that means it is.
Bloggerific! Ben Ten Form (Not)
Web in the decimal form of an irrational number like: The set of real numbers that cannot be written in the form of p q, where p and q are integers, is known as irrational numbers. Web in mathematics, an irrational number is a real number that cannot be written as a complete ratio of two integers. Web an irrational.
Decimal Terminating & Recurring are NOT irrational numbers Things
Web an irrational number is a number that cannot be written in the form of a common fraction of two integers. The first few digits look like this:. Web to decide if an integer is a rational number, we try to write it as a ratio of two integers. Web pi is a famous irrational number. Web in the decimal.
Web In Mathematics, An Irrational Number Is A Real Number That Cannot Be Written As A Complete Ratio Of Two Integers.
The decimal form of a. Irrational numbers are nonterminating and nonrepeating. So irrational number is a number that is not rational that means it is a. I verified π π and all the.
Web A Rational Number Is Of The Form , P = Numerator, Q= Denominator, Where P And Q Are Integers And Q ≠0.
Web an irrational number is a number that cannot be written in the form of a common fraction of two integers. 1 2, 11 3, 5 1, 3.25, 0.252525. Web pi is a famous irrational number. The first few digits look like this:.
Web 1 2 3 4 5 6 Rational And Irrational Numbers A Number Is Described As Rational If It Can Be Written As A Fraction (One Integer Divided By Another Integer).
¯ 3 is rational because this number can be written as the ratio of 16. Web definition of irrational numbers. Web rational numbers are numbers that can be written as a ratio of two integers. Web to decide if an integer is a rational number, we try to write it as a ratio of two integers.
Its Decimal Form Does Not Stop And Does Not Repeat.
Let's summarize a method we can use. Its decimal form does not stop and does not repeat. The set of real numbers that cannot be written in the form of p q, where p and q are integers, is known as irrational numbers. Web which statements are true for irrational numbers written in decimal form?