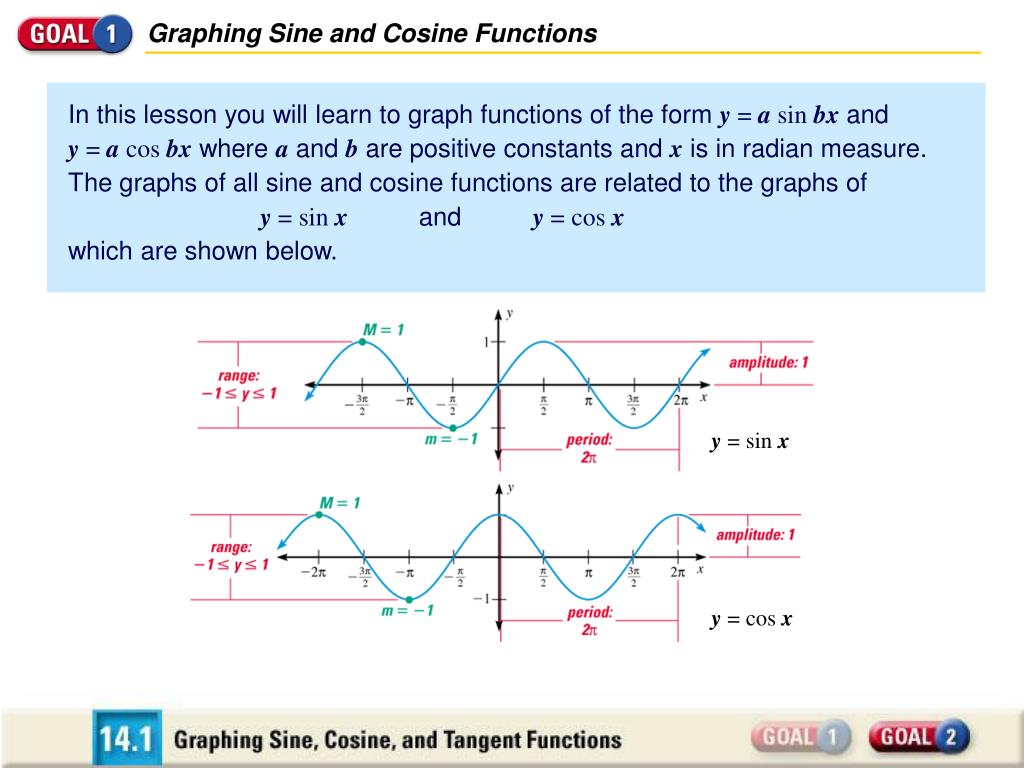
Sine And Cosine Exponential Form

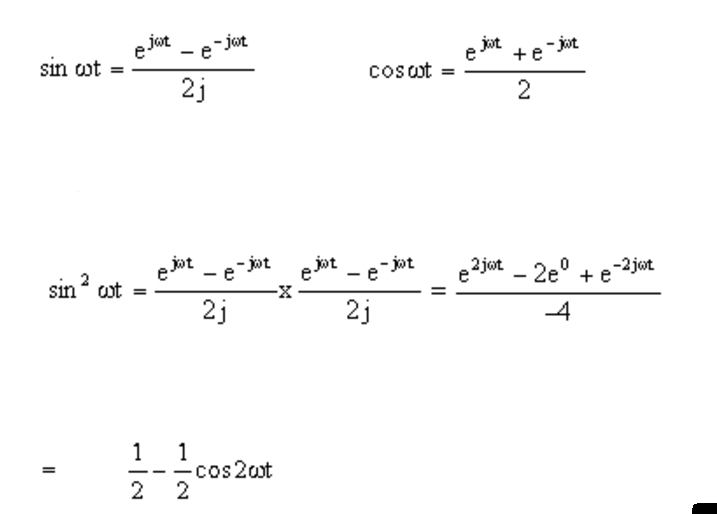
Sine And Cosine Exponential Form - As a result, the other hyperbolic functions are meromorphic in the whole complex plane. Web we can use euler’s theorem to express sine and cosine in terms of the complex exponential function as s i n c o s 𝜃 = 1 2 𝑖 𝑒 − 𝑒 , 𝜃 = 1 2 𝑒 + 𝑒. Fourier series coefficients are discussed for real signals. Web the exponential form of fourier series is presented from which the sine cosine form is derived. The sine function is one of the basic functions encountered in trigonometry (the others being the cosecant, cosine , cotangent, secant, and tangent ). (45) (46) (47) from these relations and the properties of exponential multiplication you can painlessly prove all. Web i am in the process of doing a physics problem with a differential equation that has the form: Using these formulas, we can derive further. Web today, we derive the complex exponential definitions of the sine and cosine function, using euler's formula. Web specifically, they are the inverses of the sine, cosine, tangent, cotangent, secant, and cosecant functions, [10] and are used to obtain an angle from any of the angle's.

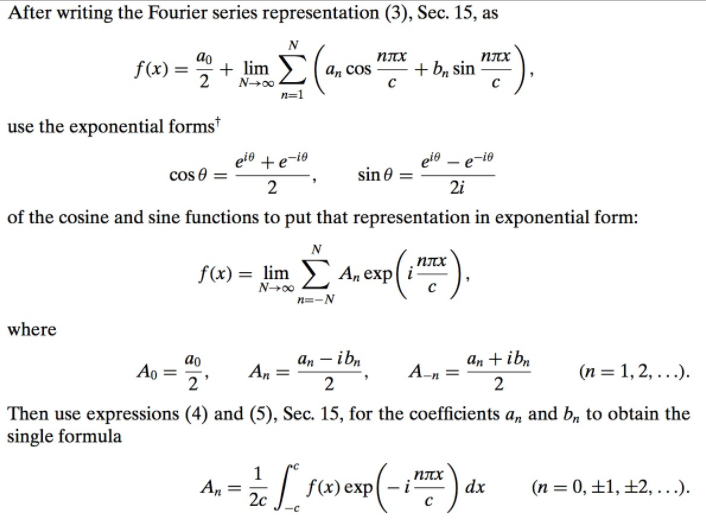
Web up to 5% cash back to represent the fourier series in concise form, the sine and cosine terms of trigonometric form, the fourier series are expressed in terms of exponential function. Web today, we derive the complex exponential definitions of the sine and cosine function, using euler's formula. As a result, the other hyperbolic functions are meromorphic in the whole complex plane. Web i am in the process of doing a physics problem with a differential equation that has the form: By thinking of the sine and cosine values as coordinates. Z cos(ax)sin(bx)dx or z sin(ax)sin(bx)dx are usually done by using the addition formulas for the cosine and sine functions. Web integrals of the form z cos(ax)cos(bx)dx; Y = acos(kx) + bsin(kx) according to my notes, this can also be written. (45) (46) (47) from these relations and the properties of exponential multiplication you can painlessly prove all. Web the exponential form of fourier series is presented from which the sine cosine form is derived.

This question does not appear to be about electronics design within the scope defined in. Y = acos(kx) + bsin(kx) according to my notes, this can also be written. (45) (46) (47) from these relations and the properties of exponential multiplication you can painlessly prove all. Web today, we derive the complex exponential definitions of the sine and cosine function, using euler's formula. Web relations between cosine, sine and exponential functions. As a result, the other hyperbolic functions are meromorphic in the whole complex plane. It is not currently accepting answers. Z cos(ax)sin(bx)dx or z sin(ax)sin(bx)dx are usually done by using the addition formulas for the cosine and sine functions. Web because we can evaluate the sine and cosine of any real number, both of these functions are defined for all real numbers. Using these formulas, we can derive further.

Relationship between sine, cosine and exponential function

As a result, the other hyperbolic functions are meromorphic in the whole complex plane. Web we can use euler’s theorem to express sine and cosine in terms of the complex exponential function as s i n c o s 𝜃 = 1 2 𝑖 𝑒 − 𝑒 , 𝜃 = 1 2 𝑒 + 𝑒. This question does not appear.

Write Equations Of Sine Functions Using Properties Calculator

Web conversion from exponential to cosine asked 7 years, 8 months ago modified 7 years, 8 months ago viewed 12k times 2 i'm trying to understand the following. Web i am in the process of doing a physics problem with a differential equation that has the form: Web the hyperbolic sine and the hyperbolic cosine are entire functions. Z cos(ax)sin(bx)dx.

PPT Graphing Sine and Cosine Functions PowerPoint Presentation, free

Web conversion from exponential to cosine asked 7 years, 8 months ago modified 7 years, 8 months ago viewed 12k times 2 i'm trying to understand the following. The sine function is one of the basic functions encountered in trigonometry (the others being the cosecant, cosine , cotangent, secant, and tangent ). Y = acos(kx) + bsin(kx) according to my.

Function For Sine Wave Between Two Exponential Cuves Mathematics

The sine function is one of the basic functions encountered in trigonometry (the others being the cosecant, cosine , cotangent, secant, and tangent ). It is not currently accepting answers. Web i am in the process of doing a physics problem with a differential equation that has the form: As a result, the other hyperbolic functions are meromorphic in the.

Basics of QPSK modulation and display of QPSK signals Electrical

Web we can use euler’s theorem to express sine and cosine in terms of the complex exponential function as s i n c o s 𝜃 = 1 2 𝑖 𝑒 − 𝑒 , 𝜃 = 1 2 𝑒 + 𝑒. The sine function is one of the basic functions encountered in trigonometry (the others being the cosecant, cosine ,.

EM to Optics 10 Converting Cos & Sine to Complex Exponentials YouTube

Web integrals of the form z cos(ax)cos(bx)dx; Web the hyperbolic sine and the hyperbolic cosine are entire functions. This question does not appear to be about electronics design within the scope defined in. Z cos(ax)sin(bx)dx or z sin(ax)sin(bx)dx are usually done by using the addition formulas for the cosine and sine functions. By thinking of the sine and cosine values.

Sine and cosine problems Math Tutoring & Exercises

Using these formulas, we can derive further. Web we can use euler’s theorem to express sine and cosine in terms of the complex exponential function as s i n c o s 𝜃 = 1 2 𝑖 𝑒 − 𝑒 , 𝜃 = 1 2 𝑒 + 𝑒. Web because we can evaluate the sine and cosine of any real.

Question Video Converting the Product of Complex Numbers in Polar Form

Web we can use euler’s theorem to express sine and cosine in terms of the complex exponential function as s i n c o s 𝜃 = 1 2 𝑖 𝑒 − 𝑒 , 𝜃 = 1 2 𝑒 + 𝑒. (45) (46) (47) from these relations and the properties of exponential multiplication you can painlessly prove all. Web the.

Other Math Archive January 29, 2018

It is not currently accepting answers. Web i am in the process of doing a physics problem with a differential equation that has the form: Web euler’s formula for complex exponentials according to euler, we should regard the complex exponential eit as related to the trigonometric functions cos(t) and. Web because we can evaluate the sine and cosine of any.

complex numbers Converting i to exponential form Mathematics

Web because we can evaluate the sine and cosine of any real number, both of these functions are defined for all real numbers. Web we can use euler’s theorem to express sine and cosine in terms of the complex exponential function as s i n c o s 𝜃 = 1 2 𝑖 𝑒 − 𝑒 , 𝜃 = 1.

Web I Am In The Process Of Doing A Physics Problem With A Differential Equation That Has The Form:

Web up to 5% cash back to represent the fourier series in concise form, the sine and cosine terms of trigonometric form, the fourier series are expressed in terms of exponential function. The sine function is one of the basic functions encountered in trigonometry (the others being the cosecant, cosine , cotangent, secant, and tangent ). Web relations between cosine, sine and exponential functions. Web today, we derive the complex exponential definitions of the sine and cosine function, using euler's formula.

Web The Exponential Form Of Fourier Series Is Presented From Which The Sine Cosine Form Is Derived.

Web we can use euler’s theorem to express sine and cosine in terms of the complex exponential function as s i n c o s 𝜃 = 1 2 𝑖 𝑒 − 𝑒 , 𝜃 = 1 2 𝑒 + 𝑒. Let be an angle measured. This question does not appear to be about electronics design within the scope defined in. It is not currently accepting answers.

Web Specifically, They Are The Inverses Of The Sine, Cosine, Tangent, Cotangent, Secant, And Cosecant Functions, [10] And Are Used To Obtain An Angle From Any Of The Angle's.

(45) (46) (47) from these relations and the properties of exponential multiplication you can painlessly prove all. As a result, the other hyperbolic functions are meromorphic in the whole complex plane. Using these formulas, we can derive further. Y = acos(kx) + bsin(kx) according to my notes, this can also be written.

By Thinking Of The Sine And Cosine Values As Coordinates.

Web the hyperbolic sine and the hyperbolic cosine are entire functions. Web because we can evaluate the sine and cosine of any real number, both of these functions are defined for all real numbers. Web integrals of the form z cos(ax)cos(bx)dx; Z cos(ax)sin(bx)dx or z sin(ax)sin(bx)dx are usually done by using the addition formulas for the cosine and sine functions.