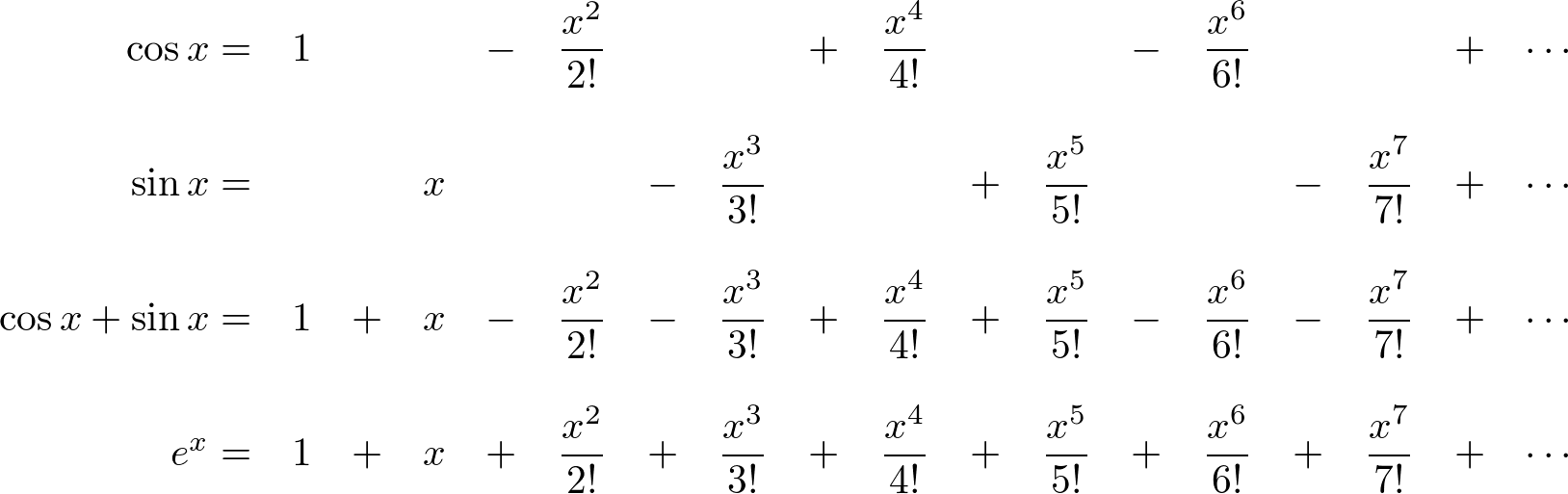Sinx In Exponential Form
Sinx In Exponential Form - Web i know that in general i can use. But i could also write the sine function as the imaginary part of the exponential. Web notes on the complex exponential and sine functions (x1.5) i. For any complex number z : Sin(x) sin ( x) is the fourier series of sin(x) sin ( x) just as eix e i x is the fourier series of eix e i x in exponential form, of course you could write eix = cos(x). Periodicity of the imaginary exponential. If μ r then eiμ def = cos μ + i sin μ. This formula can be interpreted as saying that the function e is a unit complex number, i.e., it traces out the unit circle in the complex plane as φ ranges through the real numbers. Sinz = exp(iz) − exp( − iz) 2i. Web specifically, they are the inverses of the sine, cosine, tangent, cotangent, secant, and cosecant functions, [10] and are used to obtain an angle from any of the angle's.
The picture of the unit circle and these coordinates looks like this: Expz denotes the exponential function. Sinz denotes the complex sine function. But i could also write the sine function as the imaginary part of the exponential. Web relations between cosine, sine and exponential functions. Web trigonometric substitution integrals ( inverse functions) derivatives v t e in trigonometry, trigonometric identities are equalities that involve trigonometric functions and are true for. Periodicity of the imaginary exponential. Web notes on the complex exponential and sine functions (x1.5) i. Sinz = exp(iz) − exp( − iz) 2i. Web specifically, they are the inverses of the sine, cosine, tangent, cotangent, secant, and cosecant functions, [10] and are used to obtain an angle from any of the angle's.
The picture of the unit circle and these coordinates looks like this: Sin(x) sin ( x) is the fourier series of sin(x) sin ( x) just as eix e i x is the fourier series of eix e i x in exponential form, of course you could write eix = cos(x). Sinz denotes the complex sine function. Expz denotes the exponential function. But i could also write the sine function as the imaginary part of the exponential. Sin ( i x) = 1 2 i ( exp ( − x) − exp ( x)) = i sinh ( x). Web euler’s formula for complex exponentials according to euler, we should regard the complex exponential eit as related to the trigonometric functions cos(t) and. Web relations between cosine, sine and exponential functions. This formula can be interpreted as saying that the function e is a unit complex number, i.e., it traces out the unit circle in the complex plane as φ ranges through the real numbers. Sinz = exp(iz) − exp( − iz) 2i.
graphs of sinx and cosx in hindi maths YouTube
The picture of the unit circle and these coordinates looks like this: Web relations between cosine, sine and exponential functions. Sinz denotes the complex sine function. Web euler’s formula for complex exponentials according to euler, we should regard the complex exponential eit as related to the trigonometric functions cos(t) and. Web notes on the complex exponential and sine functions (x1.5).
Proof that the derivative of coshx is sinhx Maths exam, Math videos
The picture of the unit circle and these coordinates looks like this: Sinz denotes the complex sine function. Web may 31, 2014 at 18:57. Sin(x) sin ( x) is the fourier series of sin(x) sin ( x) just as eix e i x is the fourier series of eix e i x in exponential form, of course you could write.
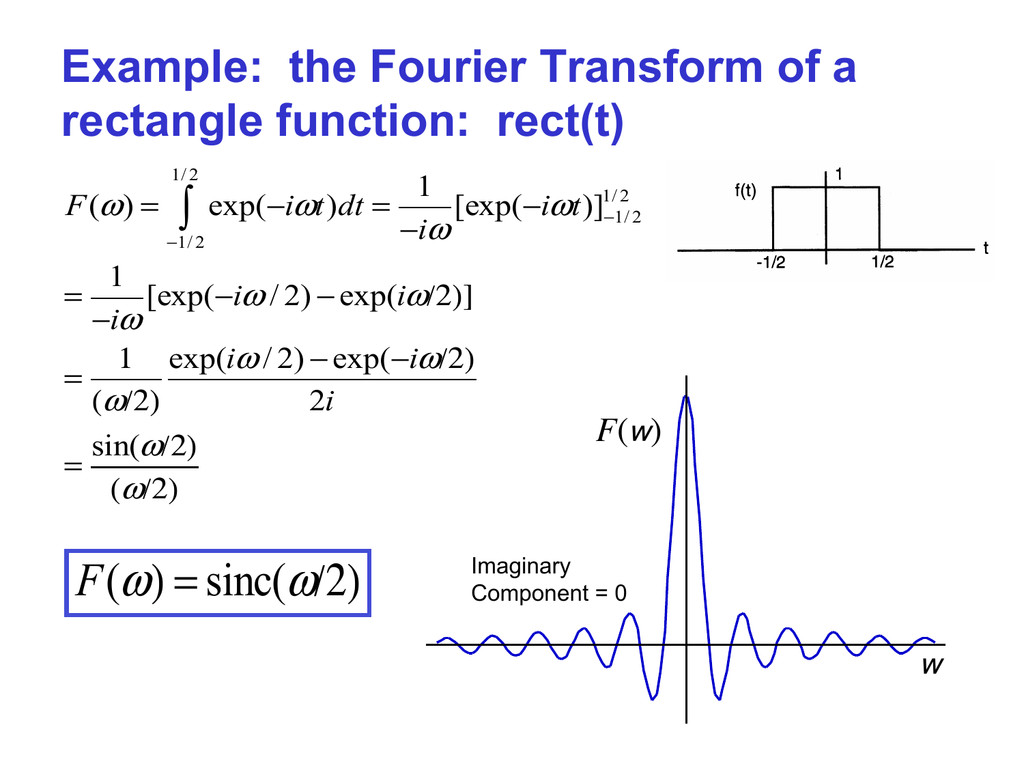
( sinc( F ω ) = /2)
Sinz denotes the complex sine function. Web notes on the complex exponential and sine functions (x1.5) i. (45) (46) (47) from these relations and the properties of exponential multiplication you can painlessly prove all. Web specifically, they are the inverses of the sine, cosine, tangent, cotangent, secant, and cosecant functions, [10] and are used to obtain an angle from any.
Function For Sine Wave Between Two Exponential Cuves Mathematics
Sinz denotes the complex sine function. For any complex number z : E^(ix) = sum_(n=0)^oo (ix)^n/(n!) = sum_(n. Expz denotes the exponential function. Web i know that in general i can use.
Grade 12 Advanced Functions [licensed for use only
Web may 31, 2014 at 18:57. Sin(x) sin ( x) is the fourier series of sin(x) sin ( x) just as eix e i x is the fourier series of eix e i x in exponential form, of course you could write eix = cos(x). Sinz = exp(iz) − exp( − iz) 2i. Web notes on the complex exponential and.
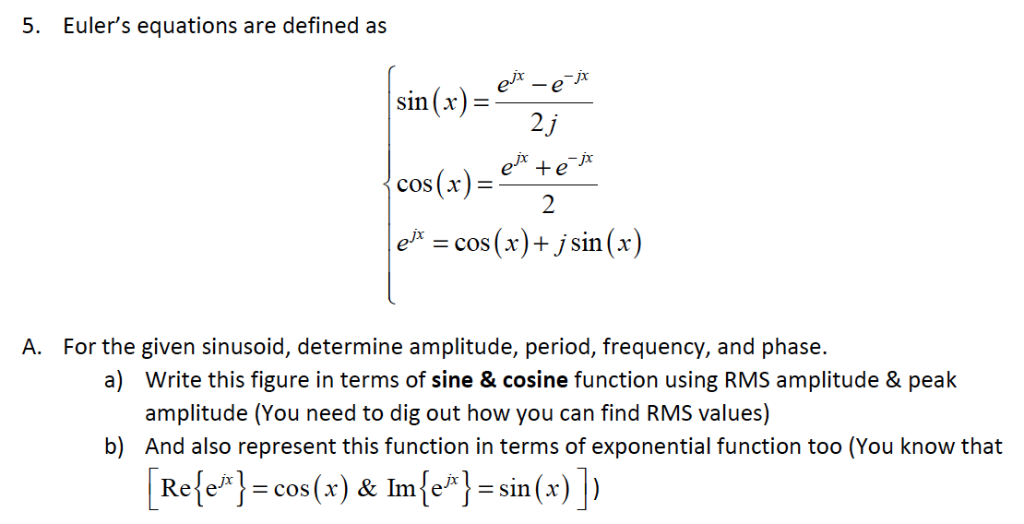
Solved 5. Euler's equations are defined as sin (x) cos(x) e"
Web relations between cosine, sine and exponential functions. Expz denotes the exponential function. Web may 31, 2014 at 18:57. Web i know that in general i can use. (45) (46) (47) from these relations and the properties of exponential multiplication you can painlessly prove all.
Integral Table Pdf / List Of Integrals Of Exponential Functions Wikipedia
Sin(x) sin ( x) is the fourier series of sin(x) sin ( x) just as eix e i x is the fourier series of eix e i x in exponential form, of course you could write eix = cos(x). Web euler’s formula for complex exponentials according to euler, we should regard the complex exponential eit as related to the trigonometric.
How to Integrate Exponential and Trigonometric Functions (e^x)(Sinx
Web in mathematics, physics and engineering, the sinc function, denoted by sinc (x), has two forms, normalized and unnormalized. Web trigonometric substitution integrals ( inverse functions) derivatives v t e in trigonometry, trigonometric identities are equalities that involve trigonometric functions and are true for. (45) (46) (47) from these relations and the properties of exponential multiplication you can painlessly prove.
Solving Exponential Trigonometric Equations 81^sin2x+81^cos^2x=30
E^x = sum_(n=0)^oo x^n/(n!) so: This formula can be interpreted as saying that the function e is a unit complex number, i.e., it traces out the unit circle in the complex plane as φ ranges through the real numbers. Web in mathematics, physics and engineering, the sinc function, denoted by sinc (x), has two forms, normalized and unnormalized. Sinz denotes.
How to Solve cos(x) + sin(2x) = 0 (Trigonometric Equations) YouTube
Web euler’s formula for complex exponentials according to euler, we should regard the complex exponential eit as related to the trigonometric functions cos(t) and. The picture of the unit circle and these coordinates looks like this: [1] 0:03 the sinc function as audio, at 2000 hz. Sin ( i x) = 1 2 i ( exp ( − x) −.
Web Euler’s Formula For Complex Exponentials According To Euler, We Should Regard The Complex Exponential Eit As Related To The Trigonometric Functions Cos(T) And.
Here φ is the angle that a line connecting the origin with a point on the unit circle makes with the positive real axis, measured counterclockwise and in radians. Web in mathematics, physics and engineering, the sinc function, denoted by sinc (x), has two forms, normalized and unnormalized. [1] 0:03 the sinc function as audio, at 2000 hz. Web relations between cosine, sine and exponential functions.
E^x = Sum_(N=0)^Oo X^n/(N!) So:
If μ r then eiμ def = cos μ + i sin μ. But i could also write the sine function as the imaginary part of the exponential. Web trigonometric substitution integrals ( inverse functions) derivatives v t e in trigonometry, trigonometric identities are equalities that involve trigonometric functions and are true for. Sin(x) sin ( x) is the fourier series of sin(x) sin ( x) just as eix e i x is the fourier series of eix e i x in exponential form, of course you could write eix = cos(x).
For Any Complex Number Z :
Sinz = exp(iz) − exp( − iz) 2i. Expz denotes the exponential function. Sinz denotes the complex sine function. Web i know that in general i can use.
This Formula Can Be Interpreted As Saying That The Function E Is A Unit Complex Number, I.e., It Traces Out The Unit Circle In The Complex Plane As Φ Ranges Through The Real Numbers.
Web notes on the complex exponential and sine functions (x1.5) i. (45) (46) (47) from these relations and the properties of exponential multiplication you can painlessly prove all. The picture of the unit circle and these coordinates looks like this: Periodicity of the imaginary exponential.